Real-space condensation in a dilute Bose gas at low temperature

Real-space condensation in a dilute Bose gas at low temperature

I. O. Kulik

Citation: Low Temperature Physics 27, 873 (2001); doi: 10.1063/1.1414580 View online: http://dx.doi.org/10.1063/1.1414580

View Table of Contents: http://aip.scitation.org/toc/ltp/27/9

Real-space condensation in a dilute Bose gas at low temperature

I. O. Kulik*

Department of Physics, Bilkent University, Bilkent, Ankara 06533, Turkey

共Submitted May 16, 2001兲

Fiz. Nizk. Temp. 27, 1179–1182共September–October 2001兲

We show with a direct numerical analysis that a dilute Bose gas in an external potential—which is chosen for simplicity as a radial parabolic well—undergoes at a certain temperature Tca

phase transition to a state supporting a macroscopic fraction of particles at the origin of the phase space共r⫽0, p⫽0兲. Quantization of particle motion in a well wipes out the sharp transition but supports a distribution of a radial particle density ␳(r) peaked at r⫽0 共a real-space condensate兲 as well as a phase-space Wigner distribution density W(r,p) peaked at r⫽0 and p⫽0 below a crossover temperature Tc* of order of Tc. A fixed-particle-number canonical

ensemble, which is a combination of the fixed-N condensate part and the fixed-␮excitation part, is suggested to resolve the difficulty of large fluctuation of the particle number (␦N⬃N) in the Bose-Einstein condensation problem treated within the orthodox grand canonical

ensemble formalism. © 2001 American Institute of Physics. 关DOI: 10.1063/1.1414580兴

The phenomenon of Bose-Einstein 共BE兲 condensation

共see textbooks, e.g., Refs. 1–3兲 manifests itself in the

forma-tion of macroscopic fracforma-tion of zero-momentum particles uniformly distributed in a coordinate space. Such transition was recently observed in laser-trapped, evaporation-cooled atomic vapors4 – 6 in magnetic traps 共see recent reviews7–9兲. We will show by a direct numerical analysis, partly similar to and sometimes overlapping with the previous theoretical works on the subject,10–13 that a Bose gas in an external confining potential condenses at low temperature to a posi-tion of minimum potential energy; the particles of that ‘‘con-densate’’ also have zero kinetic energy. Quantization of par-ticle states in a well makes the real-space condensation a continuous transition rather than a phase transition but still supports a macroscopic fraction of particles near the origin of the coordinate space below a crossover temperature T_c* which is of the order of Bose-condensation temperature T_c. Experimental realization of BE condensation implies confinement of a dilute gas within some region of space in a ‘‘trap’’ cooled by its interaction with an ‘‘optical molasses’’ created by laser irradiation14 and finally cooled to microwave-range temperature by evaporative cooling.11 Bose gas in a trap may be considered to be interacting with two thermal reservoirs, the first one representing the thermal environment 共walls, blackbody radiation at temperature T1兲 and the second one the optical molasses at temperature T2 ⰆT1. The equilibrium distribution of particles f (p, r, t) can be obtained by solving the Boltzmann kinetic equation

d f

dt⫽Iˆ1兵f其⫹Iˆ2兵f其, 共1兲

where Iˆ1 is the interaction term 共Stoss integral兲 correspond-ing to couplcorrespond-ing with a media 1, and Iˆ2, respectively, with media 2. If we choose for simplicity the relaxation time ap-proximation for Iˆ1,2,

Iˆi⫽⫺f⫺ fi ␶i

, 共2兲

then the solution for the equilibrium state will be

f⫽␶1 ⫺1_f 1 0_⫹␶ 2 ⫺1_f 2 0 ␶1⫺1⫹␶2⫺1 . 共3兲

The relaxation rate␶₂⫺1 is proportional to the laser intensity P. At large intensity, assuming ␶₂⫺1Ⰷ␶₁⫺1, Eq. 共3兲 gives f

⯝ f2 0_.

In a semiclassical approximation, the particle energy is

␧⫽ p 2 2m⫹ 1 2m⍀ 2_r2_{, 共4兲}

where the thermodynamic potential ⍀⫽⫺T ln Z, Z is the grand partition function共assuming zero spin of particles兲

Z⫽

冕

dpdr

共2␲ប兲3ln共1⫺e共␮⫺␧兲/T兲, 共5兲 where ប is Planck’s constant. The chemical potential ␮ is determined from 共5兲 to satisfy an equation

N⫽

冕

dpdr

共2␲ប兲3 1

e共␧⫺␮兲/T⫺1, 共6兲 where N is the number of particles. After integration over the directions of r and p we obtain

N⫽ 共4␲兲 2 共2␲ប兲3共2mT兲3/2

冉

2T m⍀2

冊

3/2 ⫻

冕

0 ⬁ x2dx

冕

0 ⬁ y2d y 1 ex2⫹y2⫺␨⫺1, 共7兲

where ␨⬍0 is the chemical potential in appropriate dimen-sionless units.

At low temperature, no nonzero value of ␨ can satisfy Eq. 共7兲. It therefore vanishes at a temperature T⫽Tc0 deter-mined from the condition ␨⫽0, thus giving

Tc0⫽ប⍀共N/␨共3兲兲1/3⫽0.94ប⍀N1/3, 共8兲

LOW TEMPERATURE PHYSICS VOLUME 27, NUMBER 9–10 SEPTEMBER–OCTOBER 2001

873

where␨(z) is the Riemann zeta function. Below Tc0, ␨

re-mains equal to zero with the total number of particles N0 having both r⫽0 and p⫽0 values, determined from

N0⫽

冉

1⫺ T3

T_c03

冊

N. 共9兲

Of course, the r⫽0, p⫽0 state is not allowed quantum-mechanically, and the derivation leading to Eqs. 共6兲, 共7兲 needs modification. The energy of a particle in a parabolic well, Eq.共4兲, is

␧⫽ប⍀共n1⫹n2⫹n3⫹3/2兲, ni⫽0,1... .

Then the normalization condition, Eq.共6兲, reduces to

N⫽

兺

n⫽0 ⬁ _S n ␩enx⫺1 共10兲 with Sn⫽

兺

n₁,n₂,n_3⫽0 n ␦n₁⫹n₂⫹n₃,n⫽ 1 2共n⫹1兲共n⫹2兲

and ␩⫽exp((␮0⫺␮)/T), x⫽ប⍀/T; ␮0 is the value of the chemical potential at T⫽0 (␮0⫽3/2ប⍀).

The solution of Eq. 共10兲 shows the dependence ␮(T)

共Fig. 1兲 with a crossover between almost linear dependence

above the crossover temperature T_c* and a practically zero value below that temperature. The value of T_c*is very near to Tc0at large number of particles, NⰇ1.

The particle density distribution is expressed through the sum of Hermite polynomials.15 Employing the identity for these polynomials

兺

n₁⫹n₂⫹...⫹n_r⫽nk

兿

⫽1 r _H n_k 2 _共xk兲 2nk_n k! ⫽

兺

m⫽0 n rn⫺m 1 2mm!Hm 2

冉冉

兺

k⫽1 r x_k2

冊

1/2

冊

, 共11兲

where rm⫽1 for m even and rm⫽0 for m odd, we receive by putting r⫽3 n共r兲⫽e ⫺r2 ␲3/2

兺

m⫽0 ⬁ _H m 2_共r兲 2mm! k

兺

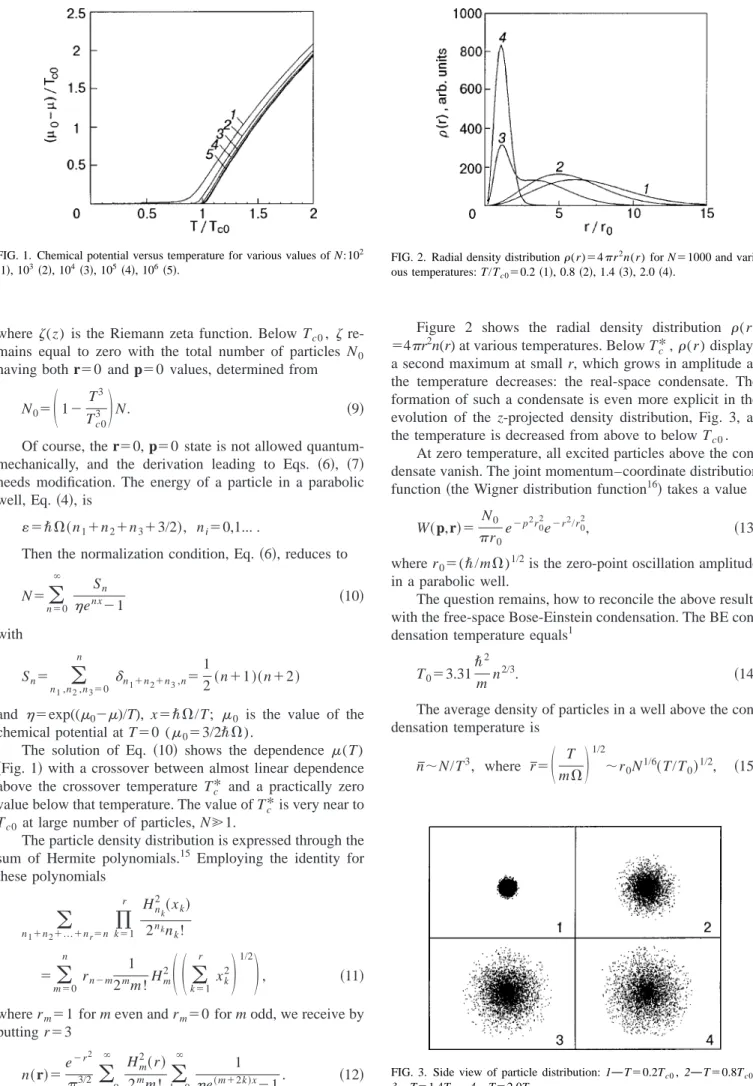
⫽0 ⬁ ₁ ␩e共m⫹2k兲x⫺1. 共12兲

Figure 2 shows the radial density distribution ␳(r)

⫽4␲r2n(r) at various temperatures. Below T_c*,␳(r) displays a second maximum at small r, which grows in amplitude as the temperature decreases: the real-space condensate. The formation of such a condensate is even more explicit in the evolution of the z-projected density distribution, Fig. 3, as the temperature is decreased from above to below Tc0.

At zero temperature, all excited particles above the con-densate vanish. The joint momentum–coordinate distribution function共the Wigner distribution function16兲 takes a value

W共p,r兲⫽ N0 ␲r0

e⫺p2r₀2_e⫺r2/r₀2_{, 共13兲} where r0⫽(ប/m⍀)1/2is the zero-point oscillation amplitude in a parabolic well.

The question remains, how to reconcile the above results with the free-space Bose-Einstein condensation. The BE con-densation temperature equals1

T0⫽3.31 ប2

mn

2/3_{. 共14兲}

The average density of particles in a well above the con-densation temperature is n ¯⬃N/T3, where r¯⫽

冉

T m⍀

冊

1/2 ⬃r0N1/6共T/T0兲1/2, 共15兲

FIG. 1. Chemical potential versus temperature for various values of N:102

共1兲, 103_{共2兲, 10}4_{共3兲, 10}5_{共4兲, 10}6_共5兲. FIG. 2. Radial density distribution␳(r)⫽4␲r 2

n(r) for N⫽1000 and

vari-ous temperatures: T/Tc0⫽0.2 共1兲, 0.8 共2兲, 1.4 共3兲, 2.0 共4兲.

FIG. 3. Side view of particle distribution: 1—T⫽0.2Tc0, 2—T⫽0.8Tc0,

3—T⫽1.4Tc0, 4—T⫽2.0Tc0.

where r¯ is a confinement radius共mean radius of the gaseous cloud兲. It is related to the minimal quantum radius r0 as r¯ ⬃r0N1/6(T/Tc)1/2. By putting T⬃Tc* as defined above, we

obtain T of the order of the BE condensation temperature

共14兲. Therefore, the phenomenon we discussed is just the BE

condensation mechanism,1except that in a trap the conden-sation occurs in both the momentum and coordinate spaces or, if we choose to explore the behavior of a dilute low-temperature Bose gas in real space, it will condense there, making up a high-density globular fraction coexisting with the spatially dispersed ‘‘excitations’’ in a region of size com-parable to the thermal confinement radius r¯.

In the grand canonical ensemble which we so far have been considering, the number of particles is not fixed. The mean square fluctuation of particle number in a state ␣ is

具

␦n_␣2

典

⫽n_␣(n_␣⫹1). In a condensate, by putting

具

n_␣⫽0

典

⫽N0 we get␮⯝␧0⫺T/N0 and

具

␦n0 2

_典

1/2_⯝N

0. This means a huge fluctuation of particle number ␦N⬃N at TⰆT0, an unrealistic property of the model.17

In a canonical ensemble, which better fits to experiments with dilute gases in traps, the average value of the conden-sate population is given by

具

n0

典

⫽ 兺nN_0⫽0 n0兺兵n_␣其⬘e⫺␤兺␣⬎0共␧␣⫺␧0兲n␣␦兺_␣⬎0n_␣, N⫺n₀ 兺nN_0⫽0兺 兵n_␣其⬘e⫺␤兺␣⬎0共␧␣⫺␧0兲n␣␦兺_␣⬎0n_␣, N⫺n₀ , 共16兲 where兵n_␣其

⬘

stands for a collection of all state numbers ex-cept n0, and␤⫽1/T. The average over such states does not fluctuate strongly and therefore can be replaced by its grand canonical value corresponding to an appropriate choice of chemical potential ␮⫽␮N⫺n₀. We thus get

具

n0

典

⬵ 兺n 0⫽0 N n0ZN⫺n₀ 兺n 0⫽0 N ZN⫺n₀ , 共17兲

where Z_n⫽e⫺␤⍀n_{, and}⍀n_{is the thermodynamic potential of}

the grand canonical ensemble.1

The quantity Zn⫽e⫺␤N is not exponentially small for a number of particles n smaller than the Bose-condensate frac-tion, n⬍N0. Therefore, we can change expression共17兲 to

具

n0

典

⬵ 兺n₀⫽N0 N _n 0e⫺␤⍀N⫺n0 兺n₀⫽N0 N _e⫺␤⍀_N⫺n 0 . 共18兲

The quantity ⍀n is strongly peaked at n⫽N0, thus giving

具

n0

典

⯝N0 and, similarly,

具

␦n0

2

_典

1/2_⬃

冑

_N

0 rather than

具

␦n₀2

典

1/2⬃N0 as in the orthodox grand canonical ensemble. Indeed, at NⰆN0共corresponding to TⰇT0兲 we obtain for the thermodynamic potential ⍀N a value ⍀N⯝⫺NT, and ZN

⯝eN_{. This agrees with the conclusion, reached in a different}

way in Ref. 12, that the thermodynamic properties of a Bose condensate in a trap with fixed total number of particles are very similar to those in the orthodox grand canonical en-semble with a fixed average number of particles. The above results are consistent with a known statement that the Bose-Einstein condensation temperature T0 is the same in the ca-nonical and in the grand caca-nonical ensembles.2

In conclusion, I hope I have met the goal of elucidating in a direct way the properties of the low-temperature state of an ideal Bose gas of finite-size, finite-particle-number sys-tems. I express my deep gratitude to Prof. B. Tanatar for stimulating discussions and help.

*Also at: B. Verkin Institute for Low Temperature Physics and Engineering of the National Academy of Sciences of Ukraine, 47 Lenin. Ave., Kharkov 61103, Ukraine.

E-mail: kulik@fen.bilkent.edu.tr

1

L. D. Landau and E. M. Lifshitz, Statistical Physics, Vol. 1, Pergamon, New York共1987兲.

2_{K. Huang, Statistical Mechanics, Wiley, New York共1987兲.}

3_{A. H. Carter, Classical and Statistical Thermodynamics, Prentice Hall,}

New Jersey共2001兲.

4

M. H. Anderson, J. R. Ensher, M. R. Matthews, C. E. Wieman, and E. A. Cornell, Science 269, 198共1995兲.

5_{K. B. Davis, M. O. Mewes, M. A. Joffe, M. R. Andrews, and W. Keterle,}

Phys. Rev. Lett. 74, 5202共1995兲.

6

W. Petrich, M. H. Anderson, J. R. Ensher, and E. A. Cornell, Phys. Rev. Lett. 74, 3352共1995兲.

7_{F. Dalfovo, S. Giorgini, L. P. Pitaevskii, and S. Stringati, Rev. Mod. Phys.}

71, 463共1999兲.

8

W. Ketterle, D. S. Dufree, and D. M. Stamper-Kurn, Making, Probing and

Understanding Bose-Einstein Condensates, Preprint xxx.lanl.gov/abs/

cond-mat/9904034共1999兲.

9_{E. A. Cornell, J. R. Ensler, and C. E. Wieman, Experiments in Dilute}

Atomic Bose-Einstein Condensation, Preprint xxx.lanl.gov/abs/cond-mat/

9903109共1999兲.

10_{N. L. Balazs and T. Bergeman, Phys. Rev. A 58, 2359共1998兲.} 11_{W. Ketterle and N. J. van Druten, Phys. Rev. A 54, 656共1996兲.} 12_{P. Navez, D. Bitouk, M. Gajda, Z. Idziaszek, and K. Rzazewski, Phys.}

Rev. Lett. 79, 1789共1997兲.

13

F. Brosens, J. T. Devreese, and L. F. Lemmens, Phys. Rev. E 55, 6795

共1997兲.

14_{Y. Castin, J. Dalibard, and C. Cohen-Tannoudji, Laser Cooling and}

Trap-ping of Neutral Atoms, in Atoms in Electromagnetic Fields, edited by C.

Cohen-Tannoudji, World Scientific, Singapore共1994兲.

15

I. S. Gradsteyn and I. M. Ryzhik, Tables of Integrals, Sums and Products, edited by A. Jeffrey, Acad. Press, New York共1994兲.

16_{E. P. Wigner, Phys. Rev. 40, 749共1932兲.} 17_{M. Fierz, Helv. Phys. Acta 29, 47共1995兲.}

This article was published in English in the original Russian journal. Repro-duced here with stylistic changes by AIP.

875